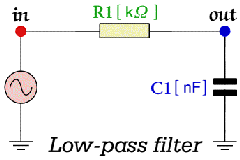
You can use the buttons provided in the above experiment to alter the values of the applied frequency and the circuit components. You should find that the circuit passes 'low' frequencies fairly well, but attenuates 'high' frequencies. Hence it is useful as a filter to block any unwanted high frequency components of a complex signal whilst passing the lower frequencies.
Of course, the meanings of 'low' and 'high' frequencies are relative. In this case they depend upon the filter's Time Constant which is normally represented by the Greek letter 'tau' and has a value
The action of the circuit can also be described in terms of a related quantity, the Turn Over Frequency, f0, which has a value
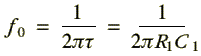
The circuit's behaviour can be understood as arising due to the finite time taken to change the capacitor's charge when we alter the applied input voltage. As a result, the output voltage (i.e. the voltage across the capacitor) tends to 'lag' behind changes in the input. The more quickly we alter the input (i.e. the higher the applied frequency) the more this lag becomes apparent and the less chance we give the output to respond. Because of this behaviour, we find that the output sinusoid is out of phase with the input. We can therefore define the circuit's overall behaviour when we apply a sinewave in terms of two quantities
The Voltage Gain:

The Phase Delay:
The voltage gain tells the relative size of the output voltage compared to the input voltage. As is often the case the word 'gain' is misleading as the output turns out to be smaller than the input!
Try using the above experimental system to collect results and plot a graph of how the voltage gain, Av, (and the phase delay) depend upon the input frequency and check that your result agrees with the above formulae. You should find that frequencies much lower than f0 are passed almost unattenuated (i.e. Av approaches 100%), but frequencies much higher than f0 are strongly attenuated (i.e. Av approaches 0%). Once you are happy that this is correct try using the experimental system to choose circuit values that give a turn over frequency of around, say, 2kHz. Then, if you get a chance, try building a real circuit and see if it behaves like the computer experiment.
Compare the results you get from this circuit with those produced by a similar High-Pass Filter arrangement.
More generally, you can use this page to 'design' a low pass filter. However, remember that, like most computer experiments, it may not be perfectly accurate, so your real results may differ a little bit!